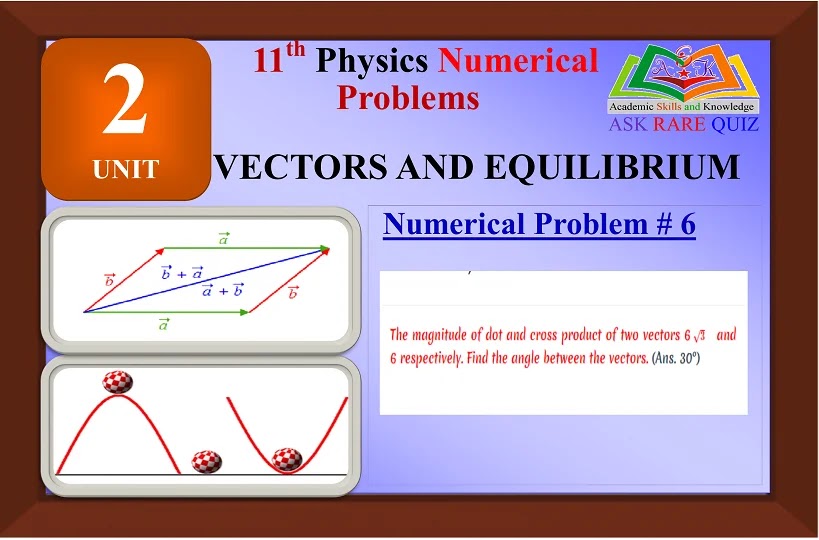
The magnitude of the dot and cross product of two vectors 6`\ sqrt{3}`   and 6 respectively. Find the angle between the vectors. (Ans. 30°)


Given:

Let First vector is `\vec {A}` and second vector is `\vec {B}` then according to the given data

|`\vec {A}` . `\vec {B}`|= 6`\sqrt {3}`   ---------(1)
|`\vec {A}` ｘ `\vec {B}`|= 6  -------------(2)
 

To Find:

Angle between two vectors `\vec {A}` and `\vec {B}`  = θ =  ?

Solution:


In order to find θ between the two vectors, we will divide equation (2) by equation (1)

`\ frac {|vec A ｘ vec B|}{|vec A . vec B|}` = `\ frac {6}{6 sqrt 3}`

or 

We know that

|`\vec {A}` . `\vec {B}`|=A B cos θ 
and
|`\vec {A}` ｘ `\vec {B}`|= A B sin θ 

So,


`\ frac {A B sin θ}{A B cos θ}` = `\ frac {6}{6 sqrt 3}`

Or

`\ frac {sin θ}{cos θ}` = `\ frac {1}{sqrt 3}`

by simplifying 

tan θ = `\ frac {1}{sqrt 3}`                               [ `\ frac {sin θ}{cos θ}` = tan θ ]

Or

θ = tan⁻¹ `\ frac {1}{sqrt 3}` 

θ = 30°  ------------------Ans

Thus the angle between two vectors `\vec {A}` and `\vec {B}`  = θ =  30°


************************************

************************************

Shortcut Links For 


1. Website for School and College Level Physics   
2. Website for School and College Level Mathematics  
3. Website for Single National Curriculum Pakistan - All Subjects Notes 

© 2022-Onwards by Academic Skills and Knowledge (ASK)     

Note:  Write me in the comment box below for any query and also Share this information with your class-fellows and friends.